At What Interest Rate Compounded Continuously Must Money Be Invested To Double In 3 Years

2.1 Exponential and Logarithmic Function Applications

Pre-Class:

• Take notes on the videos and readings (use the infinite below).
• Work and cheque trouble #1 in the 2.1 NOTES department.
• Complete the 2.ane Pre-Class Quiz.

Introduction

Exponential functions occur frequently in scientific discipline and business and are normally used in compound interest applications.

• The value of a \$1000 investment returning 8% involvement compounded monthly after 12 years would be calculated using the formula $$A=P\left(one+\frac rn\correct)^{nt},$$

  where:

• A is the final corporeality in the account.
• P is the master.
• r is the interest rate.
• north is the number of compounding periods per yr.
• t is the number of years.


• The compounding frequency has a significant touch on the terminal amount of money (either saved or owed).

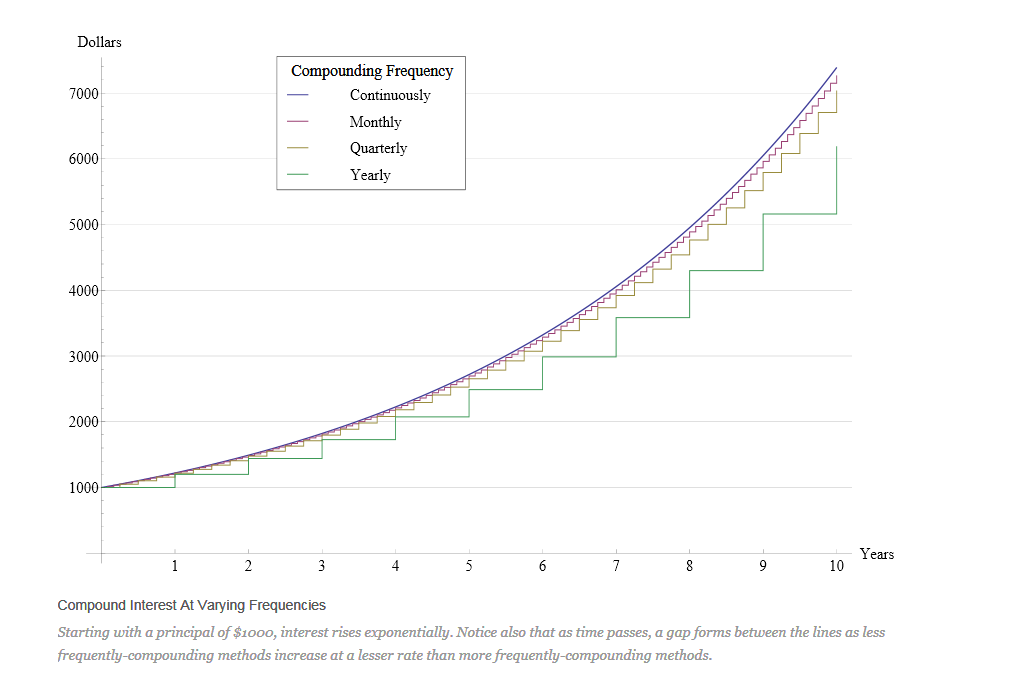
Notes

Compounding Frequency

• Yearly: $$A=1000(1+\frac{.08}1)^1=1080$$
• Quarterly: $$A=1000(i+\frac{.08}4)^iv=1082.43$$
• Monthly: $$A=1000(1+\frac{.08}{12})^{12}=1083$$
• Daily: $$A=1000(1+\frac{.08}{365})^{365}=1083.28$$
• Continuously (at every instant): $$A=thousand \cdot~\underset{n\to \infty }{\mathop{\lim }}\,{{\left( i+\frac{.08}{n} \right)}^{n}} =1083.29$$

Our focus volition be on continuous compounding:

• What is due east?
• Irrational number (similar to $\pi $ )
• ii.718281828459…..
• Like $\pi $, due east occurs oftentimes in natural phenomena
  • Growth of bacterial cultures
  • Disuse of a radioactive substance
• Formal definition of east: $$e=~\underset{n\to \infty }{\mathop{\lim }}\,{{\left( ane+\frac{i}{north} \right)}^{north}}$$ $$\approx ~~2.718281829$$

---

Notes

Continuous Compounding Formula (appreciation and depreciation): $$A = P{e^{rt}}$$

CONTINUOUS COMPOUND INTEREST: Round all answers to two decimal places.

1. Hometown Bank offers a CD that earns ane.58% compounded continuously. If \$10,000 is invested in this CD, how much will information technology be worth in 3 years?

   $A = 10,000{e^{.0158(iii)}}$

   $A = \$ 10,485.41$

   The account will be worth approximately \$$10,485.41$ in three years.

2. Hometown Bank offers a CD that earns i.58% compounded continuously. If \$10,000 is invested in this CD, how long will it accept the account to exist worth $11,000?

   $11,000 = 10,000{e^{.0158t}}$

   $\frac{{11,000}}{{10,000}} = \frac{{ten,000{east^{.0158t}}}}{{10,000}}$

   $\frac{{11}}{{10}} = {e^{.0158t}}$

   $\ln \frac{{11}}{{10}} = \ln {e^{.0158t}}$

   $\ln \frac{{11}}{{10}} = .0158t$

   $\frac{{\ln \frac{{xi}}{{ten}}}}{{.0158}} = \frac{{.0158t}}{{.0158}}$

   $six.03 = t$

   Information technology will have approximately six.03 years for the account to be worth $11,000.

3. Doubling Time: How long will it take money to double if information technology is invested at 5% compounded continuously?

   $A = P{east^{rt}}$

   $2 = one{e^{.05t}}$

   $2 = {e^{0.05t}}$

   $\ln 2 = \ln {e^{0.05t}}$

   $\ln 2 = 0.05t$

   $\frac{{\ln 2}}{{0.05}} = t$

   $t = thirteen.86$

   Information technology will take approximately xiii.86 years for the initial investment to double.

4. Doubling Rate: At what nominal rate compounded continuously must money exist invested to double in 8 years?

   $A = P{east^{rt}}$

   $two = one{e^{r(8)}}$

   $2 = {eastward^{8r}}$

   $\ln 2 = \ln {due east^{8r}}$

   $\ln 2 = 8r$

   $\frac{{\ln 2}}{8} = r$

   $0.0866 = r$

   In order for the initial investment to double in 8 years, the money must be invested in an business relationship with a nominal rate of approximately 8.vii% compounded continuously.

5. How long will information technology take money to triple if it is invested at x.5% compounded continuously?

   $A = P{e^{rt}}$

   $3 = 1{e^{.105t}}$

   $\ln three = \ln {due east^{.105t}}$

   $\ln iii = .105t$

   $\frac{{\ln iii}}{{.105}} = t$

   $10.46=t$

   It will take approximately 10.46 years for the initial investment to triple.

6. Radioactive decay: A mathematical model for the decay of radioactive substances is given by $$Q = {Q_0}\;{eastward^{rt}}.$$ The continuous compound rate of decay of carbon-14 per year is $r = -0.0001238.$ How long will it accept a certain amount of carbon-14 to decay to half the original amount?

   $\frac{one}{ii} = 1{eastward^{ - 0.0001238t}}$

   $\ln .v = \ln {e^{ - 0.0001238t}}$

   $\ln .five = - 0.0001238t$

   $\frac{{\ln .5}}{{ - 0.000128}} = t$

   $t = 5598.93$

   It will take approximately 5598.93 years for the carbon-14 to disuse to one-half the original amount.

7. The estimated resale value R (in dollars) of a company automobile after t years is given by: $$R(t) = 20000{(0.86)^t}.$$ What will exist the resale value of the motorcar afterwards two years? How long volition it take the motorcar to depreciate to half the original value?

   $R(2) = twenty,000{(0.86)^2} = \$ fourteen,792$

   The resale value of the car later two years volition be $14.792.

   $\frac{{10,000}}{{20,000}} = \frac{{20,000{{(0.86)}^t}}}{{20,000}}$

   $.5 = {0.86^t}$

   $\ln .5 = \ln {0.86^t}$

   $\ln .5 = t\ln 0.86$

   $\frac{{\ln .five}}{{\ln 0.86}} = \frac{{t\ln 0.86}}{{\ln 0.86}}$

   $\frac{{\ln .5}}{{\ln 0.86}} = t$

   $t = four.5957$

   It will take approximately 4.6 years for the car to depreciate to half its original value.

2.1 The Constant e and Natural Log Applications

Homework

Answer the following questions. Show all of your work. Round to two decimal places.

1. If you invested $1,000 in an account paying an annual pct rate (quoted rate) of two%, compounded continuously, how much would you have in your account at the cease of

   1. ane year

      $A = 1000{e^{.02(i)}} = 1020.20$

      At the end of i year, in that location will exist $1020.20 in the account.

   2. 10 years

      $A = thou{east^{.02(10)}} = 1221.40$

      At the end of x years, there volition be $1221.40 in the account.

   3. 20 years

      $A = 1000{east^{.02(xx)}} = 1491.82$

      At the end of xx years, there will be $1491.82 in the account.

   4. 50 years

      $A = 1000{east^{.02(50)}} = 2718.28$

      At the end of fifty years, at that place will be $2718.28 in the account.

2. A $one,000 investment is made in a trust fund at an annual percentage charge per unit of 12%, compounded continuously. How long volition it accept the investment to

   1. Double

      $2000 = thou{e^{0.12t}}$

      $two = {e^{0.12t}}$

      $\ln 2 = \ln {e^{0.12t}}$

      $\ln 2 = 0.12t$

      $\frac{{\ln 2}}{{0.12}} = t$

      $t = five.78$

      The investment will double in approximately 5.78 years.

   2. Triple

      $3000 = grand{due east^{0.12t}}\quad$

      $iii = {e^{0.12t}}$

      $\ln three = \ln {east^{0.12t}}$

      $\ln 3 = 0.12t$

      $\frac{{\ln 3}}{{0.12}} = t$

      $t = 9.xvi$

      The investment will triple in approximately 9.xvi years.

3. If $500 is invested in an account which offers 0.75%, compounded continuously find:

   1. The amount A in the account after t years.

      $A = 500{e^{.0075t}}$

   2. Determine how much is in the business relationship later on v years, ten years, 30 years, and 35 years.

      $A(v) = 500{east^{.0075(v)}}=519.11$

      Afterwards 5 years, $519.11 will be in the account.

      $A(x) = 500{e^{.0075(10)}}=538.94$

      Later on 10 years, $538.94 volition be in the business relationship.

      $A(30) = 500{e^{.0075(xxx)}}=626.16$

      Afterwards xxx years, $626.16 volition be in the business relationship.

      $A(35) = 500{east^{.0075(35)}}=650.09$

      After 35 years, $650.09 will exist in the account.

   3. Make up one's mind how long it will take for the initial investment to double.

      $grand = 500{e^{.0075t}}$

      $two = {due east^{.0075t}}$

      $\ln ii = \ln {e^{.0075t}}$

      $\ln 2 = .0075t$

      $\frac{{\ln 2}}{{.0075}} = t$

      Information technology volition take approximately 92.42 years for the initial investment to double.

   4. Find and interpret the average rate of change of the corporeality in the account from the end of the quaternary year (t=four) to the cease of the fifth year (t=5).

      4th yr $A(four) = 500{e^{.0075(4)}} = \$515.23$

      5th year $A(5) = 500{e^{.0075(5)}} = \$519.11$

      $\frac{A(5)-A(iv)}{5-iv}=\frac{519.11-515.23}{1}=3.88$

      The balance in the account is increasing by an boilerplate of $3.88 per year.

4. If $5000 is invested in an business relationship which offers two.125%, compounded continuously, find:

   1. The corporeality A in the business relationship later t years.

      $A(t) = 5000{e^{.02125t}}$

   2. Determine how much is in the business relationship later on 5 years, 10 years, 30 years, and 35 years.

      $A(5) = 5000{e^{.02125(five)}} = 5560.50$

      After five years, $5560.50 volition be in the account.

      $A(10) = 5000{e^{.02125(10)}} = 6183.83$

      Afterward 10 years, $6183.83 will exist in the account.

      $A(30) = 5000{e^{.02125(30)}} = 9458.73$

      After 30 years, $9458.73 volition be in the account.

      $A(35) = 5000{e^{.02125(35)}} =10519.05$

      Later 35 years, $10519.05 will exist in the account.

   3. Determine how long it volition accept for the initial investment to double.

      $10,000 = 5000{eastward^{.02125t}}$

      $2 = {e^{.02125t}}$

      $\ln two = \ln {due east^{.02125t}}$

      $\ln 2 = .02125t$

      $\frac{{\ln 2}}{{.02125}} = t$

      Information technology will accept approximately 32.62 years for the initial investment to double.

   4. Find and interpret the average charge per unit of change of the amount in the account from the cease of the fourth year (t=four) to the end of the fifth year (t=5).

      $A(4) = 5000{e^{.02125(four)}}$

      $A(iv) = \$ 5443.59$

      $A(five) = 5000{e^{.02125(5)}}$

      $A(v) = \$ 5560.50$

      $\frac{A(v)-A(4)}{5-4}=\frac{\displaystyle5560.l-5443.59}{5-4}=\frac{\displaystyle116.91}ane=116.91$

      The balance in the account is increasing past an average of $116.91 per year.

5. How much coin needs to be invested now to obtain \$5000 in 10 years if the interest rate in a CD is 2.25%, compounded continuously?

   $A = P{eastward^{rt}}$

   $5000 = P{east^{0.0225(10)}}$

   $5000 = P{east^{0.225}}$

   $\frac{{5000}}{{{eastward^{0.225}}}} = \frac{{P{e^{0.225}}}}{{{east^{0.225}}}}$

   $\$ 3992.58 = P$

   \$3992.58 needs to be invested now, in order to have $5000 in 10 years.

6. A mathematical model for depreciation of a car is given past $A = P{(one-r)^t}$, where A is defined every bit the value of the car after t years, P is defined as the original value of the machine, and r is the rate of depreciation per year. The toll of a new car is $32,000. It depreciates at a rate of xv% per twelvemonth. This means that it loses fifteen% of its value each yr.

   1. Find the formula that gives the value of the motorcar in terms of time.

      $A = 32,000{\left( {i-0.15} \correct)^t}$

      $A = 32,000{\left( {0.85} \right)^t}$

   2. Find the value of the motorcar when information technology is four years old.

      $A = 32,000{\left( {.85} \right)^four}$

      $A = xvi,704.twenty$

      The car is worth approximately $xvi,704.20 when it is 4 years old.

7. A mathematical model for depreciation of an ATV (all-terrain vehicle) is given by $A = P{(ane-r)^t}$, where A is defined as the value of the vehicle after t years, P is defined as the original value of the vehicle, and r is the charge per unit of depreciation per yr. The price of a new ATV (all-terrain vehicle) is \$7200. It depreciates at xviii% per yr.

   1. Find the formula that gives the value of the ATV in terms of time.

      $A = 7200{\left( {1-0.18} \right)^t}$

      $A = 7200{\left( {.82} \right)^t}$

   2. Find the value of the ATV when it is ten years former.

      $A = 7200{\left( {.82} \right)^{x}}$

      $A = 989.63$

      The value of the ATV when it is x years former will be $989.63.

8. Michigan's population is declining at a charge per unit of 0.5% per twelvemonth. In 2004, the state had a population of 10,112,620.

   1. Write a function to express this situation.

      $y = x,112,620{\left( {.995} \right)^t}$

   2. If this charge per unit continues, what will the population be in 2012?

      $y = 10,112,620{\left( {.995} \correct)^eight}$

      In 2012 the population of Michigan will be approximately nine,715,124 people.

   3. When will the population of Michigan achieve 9,900,000?

      $9,900,000 = 10,112,620{\left( {.995} \right)^t}$

      $\frac{{9,900,000}}{{10,112,620}} = {.995^t}$

      $\ln (\frac{{9,900,000}}{{ten,112,620}}) = \ln {.995^t}$

      $\ln (\frac{{9,900,000}}{{ten,112,620}}) = t\;\ln .995$

      $\frac{{\ln (\frac{{9,900,000}}{{10,112,60}})}}{{\ln .995}} = t$

      $t = 4.24$ years

      The population of Michigan will exist 9,900,000 people in March of 2008.

   4. What was the population in the year 2000, according to this model?

      $y = x,112,620{\left( {.995} \right)^{ - 4}}$

      $y = 10,317,426.06$

      Co-ordinate to this model, the population of Michigan in 2000 was 10,317,426 people.

   https://sccmath.files.wordpress.com/2012/01/scc_open_source_intermediate_algebra.pdf

Source: https://psccmath.github.io/math1830/u2s1.html

Posted by: griggsnour1949.blogspot.com

Share this post